


 Disclaimer statement from PNL
Disclaimer statement from PNL
May 1994
The purpose of this paper is to examine the impact of advanced energy technologies on greenhouse gas emissions, atmospheric composition, and climate change. This analysis requires tools which cover both economic and bio-geophysical relationships. Our to ol, the Global Change Assessment Model (GCAM), is an integrated set of models that address complementary facets of the problem. We rely on the Edmonds-Reilly-Barns Model (ERB) for energy related greenhouse gas emissions. Other emissions trajectories, part icularly land-use related emissions, are taken from the IPCC, IS92 scenarios. Atmospheric composition, radiative forcing, global mean temperature change, and sea level rise are developed following Wigley and Raper (1992).
Energy-Related Emissions
We have used the ERB, version 4.15, to model energy-economy-greenhouse emissions relationships. The ERB is a well-documented (Edmonds and Reilly, 1985; Edmonds et al., 1986), frequently used, long-term model of global energy and fossil fuel greenhouse ga s emissions. The model can be thought of as consisting of four parts: supply, demand, energy balance, and greenhouse gas emissions. The first two modules determine the supply of and demand for each of six major primary energy categories in each of nine gl obal regions. The energy balance module ensures model equilibrium in each global fuel market. (Primary electricity is assumed to be untraded; thus supply and demand balance in each region.) The greenhouse gas emissions module is a set of three post-proce ssors which calculate the energy-related emissions of carbon dioxide (CO2), nitrous oxide (N2O), and methane (CH4). The original version of the model is documented in Edmonds and Reilly (1985), while major revisions are discussed in Edmonds et al. (1986). The model is currently configured to develop scenarios for benchmark years: 1990, 2005, 2020, 2035, 2050, 2065, 2080, and 2095.
Energy demand for each of the six major fuel types is developed for each of the nine regions. Five major exogenous inputs determine energy demand: population; labor productivity; exogenous energy end-use intensity; energy prices; and energy taxes, subsidi es, and tariffs.
The model calculates base GNP directly as a product of labor force and labor productivity. An estimate of base GNP for each region is used both as a proxy for the overall level of economic activity and as an index of income. The base GNP is, in turn, modi fied within the model to be consistent with energy-economy interactions. The GNP feedback elasticity is regional, allowing the model to distinguish energy supply dominant regions, such as the Mideast, where energy prices and GNP are positively related, fr om the rest of the world where the relationship is inverse.
The exogenous end-use energy-intensity improvement parameter is a time-dependent index of energy productivity. It measures the annual rate of growth of energy productivity that would continue independent of such other factors as energy prices and real inc ome changes. In the past, technological progress and other non-price factors have had an important influence on energy use in the manufacturing sector of advanced economies. Including an exogenous end-use energy-intensity improvement parameter allows scen arios to be developed that incorporate either continued improvements or technological stagnation assumptions as an integral part of these scenarios.
The final major energy factor influencing demand is energy prices. Each region has a unique set of energy prices derived from world prices (determined in the energy balance component of the model) and region-specific taxes and tariffs. The model can be mo dified to accommodate nontrading regions for any fuel or set of fuels. The model assumes that regions do not trade solar, nuclear, or hydroelectric power, but all regions trade fossil fuels.
The energy-demand module performs two functions: it establishes the demand of energy, and its services, and it maintains a set of energy flow accounts for each region. Oil and gas are transformed into secondary liquids and gases that are used either dire ctly in end-use sectors or indirectly as electricity. Hydro, nuclear, and solar electric or fusion are accounted for directly as electricity. Nonelectric solar energy is included with conservation technologies as a reduction in the demand for marketed fue ls.
The four secondary fuels are consumed to produce energy services. In each region of the model, energy is consumed by three end-use sectors: residential/commercial, industrial, and transportation.
The demand for energy services in each region's end-use sectors is determined by the cost of providing these services and by the levels of income and population. The mix of secondary fuels used to provide these services is determined by the relative costs of providing these services using each alternative fuel. The demand of fuels to provide electric power is then determined by the relative costs of production, as is the share of oil and gas transformed from coal and biomass.
Energy supply is disaggregated into two categories, renewable and non-renewable. Energy supply from all fossil fuels is related directly to the resource base by grade, the cost of production (both technical and environmental) and to the historical produc tion capacity. The introduction of a graded resource base for fossil fuel (and nuclear) supply allows the model to explicitly test the importance of fossil fuel resource constraints as well as to represent fuels such as shale oil, in which only small amou nts are likely available at low costs but for which large amounts are potentially available at high cost.
Note here that nuclear is treated in the same category as fossil fuels. Nuclear power is constrained by a resource base as long as light-water reactors are the dominant producers of power. Breeder reactors, by producing more fuel than they consume, are mo deled as an essentially unlimited source of fuel that is available at higher cost.
A rate of technological change is also introduced on the supply side. This rate varies by fuel and is expected to be both higher and less certain for emerging technologies.
The supply and demand modules each generate energy supply and demand estimates based on exogenous input assumptions and energy prices. If energy supply and demand match when summed across all trading regions in each group for each fuel, then the global en ergy system balances. Such a result is unlikely at an arbitrary set of energy prices. The energy balance component of the model is a set of rules for choosing energy prices which, on successive attempts, bring supply and demand nearer a system-wide balanc e. Successive energy price vectors are chosen until energy markets balance within a prespecifed bound.
Given the solution of the energy balance component of the model, greenhouse gas emissions for CO2, CH4, and N2O are calculated by applying emissions coefficients. Emissions coefficients for CO2 are as follows:
* liquids 19.2 TgC/EJModern biomass is treated as if its carbon absorption occurred in the year of release. This approximation can either underestimate or overestimate actual net annual fluxes depending upon whether the underlying stock of biomass is either expanding or contr acting. (See Edmonds and Barns 1990).
* gases 13.7 TgC/EJ
* solids 23.8 TgC/EJ
* carbonate rock mining 27.9 TgC/EJ
Atmosphere, Climate and Sea Level
The analysis of atmospheric composition, climate change, and sea level rise uses the MAGICC model following Wigley and Raper (1992).
The concentration of CO2 in the atmosphere is determined using a reduced form carbon cycle model. The model is balanced. That is, the model reproduces current atmospheric concentrations in a manner which does not resort to directly pairing emission sourc es and sinks. Two sink terms are considered, ocean and terrestrial. The ocean sink employs a convolution integral representation, based on Maier-Reimer and Hasselmann (1987). The terrestrial sink is modeled as four linked boxes. An important feature of th e model is that it provides a pathway by which atmospheric CO2 concentrations affect terrestrial carbon storage. This pathway allows the carbon cycle to be balanced, though it should be noted that this mechanism is a gross oversimplification of what is cu rrently known regarding the carbon cycle, and great uncertainty remains as to the disposition of anthropogenic emissions. (See for example, Wisniewski and Lugo 1992 and IPCC 1992.)
The atmospheric concentration of CH4, N2O, and the halocarbons are determined using a mass balance equation of the form:
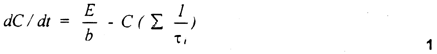
where
E = the emission rate b = a units conversion term C = the concentration, and T = the removal rate for the each sink.
Methane has two sinks: atmospheric chemical reactions and soils. For nitrous oxide and the halocarbons only the atmospheric sink is considered. It is well known that atmospheric sink rates are not constant. For methane, the availability of hydroxyl radica ls is a governing factor which in turn depends on the concentration of CH4 and the emission rates for carbon monoxide (CO), oxides of nitrogen (NOx), and volatile organic compounds (VOCs). The model explicitly considers the effect of CO, NOx, and VOC emis sions on the atmospheric removal rate.
Sulphur emissions are short lived, but their effect on climate is thought to be significant. Unlike the "greenhouse" gases, they exert a cooling effect. Because of their short lifetimes, no atmospheric stock model is needed.
Radiative forcing varies by gas. Carbon dioxide effects on radiative forcing are given by

The changes in radiative forcing associated with methane and nitrous oxide are computed as per Shine et al (1990). These methods consider direct and indirect effects on radiative forcing, as well as the effects of absorption band overlaps.
The radiative forcing associated with halocarbons has two components, a direct component and an indirect ozone component. The direct component derives from the direct warming properties of halocarbon molecules, while the indirect ozone effect takes into a ccount the cooling associated with the destruction of ozone subsequent to the dissociation of the halocarbons. The indirect ozone effect is computed as follows:

The direct effect is given by

The total effect is the sum of the direct and ozone effects.
The presence of sulphate aerosols in the atmosphere is presently felt to have a strong local cooling effect. This effect is manifest through three pathways: scattering and absorption of shortwave (solar) radiation effects, cloud reflectivity effects, and cloud persistence effects (IPCC, 1992). The effect on radiative forcing is computed as

where E is the emissions rate and Eo is the initial emissions rate.
The change in global mean temperature depends on the sum of the changes in radiative forcing, climate sensitivity, and ocean thermal inertia. The climate sensitivity is reflected by the change in global mean temperature associated with a doubling of the p reindustrial concentration of atmospheric CO2, after direct and feedback effects (for example, water vapor, ice albedo, and clouds) are taken into account. The most commonly cited range of climate sensitivity is 1.5 to 4.5 deg. C, with a "best guess" valu e of 2.5 deg. C. Ocean thermal lag is computed using an upwelling-diffusion model. The model in turn depends critically on parameters for mixed-layer depth, oceanic vertical diffusivity, the upwelling rate, and the temperature change of high-latitude sink ing water relative to the global-mean change.
Sea level rise is computed as the sum of two terms: thermal expansion and meltwater runoff (Wigley and Raper, 1992). Thermal expansion is computed from the oceanic upwelling-diffusion model referenced above. Meltwater is the sum of contributions from thre e sources: small glaciers, Greenland, and Antarctica. These in turn are driven by equilibrium temperature change.
Edmonds, J. and Barns, D.W. 1992. "Factors Affecting the Long-term Cost of Global Fossil Fuel CO2 Emissions Reductions," International Journal of Global Energy Issues,4(3): 140-166.
Edmonds, J. and Reilly, J. 1985. Global Energy: Assessing the Future, Oxford University Press, New York.
Edmonds. J.A., Reilly, J.M., Gardner, R.H., and Brenkert, A. 1986. Uncertainty in Future Global Energy Use and Fossil Fuel C02 Emissions 1975 to 2075. TR036, DO3/NBB-0081 Dist. Category UC-11, National Technical Information Service, U.S. Department of Commerce. Springfield Virginia 22161.
Grubb. M., J. Edmonds, P. ten Brink, and M. Morrison. 1993. "The Costs of Limiting Fossil-Fuel CO2 Emissions: A Survey and Analysis," Annual Review of Energy and Environment. 18:397 478.
IPCC (Intergovernmental Panel on Climate Change), 1992. Climate Change 1992: The Supplementary Report to the IPCC Scientific Assessment. J.T. Houghton. B.A. Callander and S.K. Varney (eds.), Cambridge University Press, Cambridge, United Kingdom.
Johansson T.B., H. Kelly, A.N. Reddy, R.H. Williams, eds. 1993. Renewables for Fuels and Electricity. The United Nations Solar Energy Group for Environment and Development, n.d.
Maier-Reimer, E., and Hasselmann, K. 1987. "Transport and Storage of CO2 in the Ocean: An Inorganic Ocean-Circulation Carbon Cycle Model," Climate Dynamics. 2:63-90.
Shine. K.P., Derwent, R.G., Wuebbles, D.J. and Morcrette. J.-J. 1990. The IPCC Scientific Assessment. (eds. Houghton, J.T., Jenkins, G.J. and Ephraums, J.J.):41-68. Cambridge University Press, London.
Wigley, T.M.L. and S.C.B. Raper. 1987. "Thermal expansion of seawater associated with global warming." Nature. 330:127-131.
Wigley, T.M.L., and S.C.B. Raper. 1992. "Implications for Climate and Sea Level of Revised IPCC Emissions Scenarios." Nature. Vol. 357.
Williams, R.H. 1994a, Biomass Energy Conversion Technologies for Large-scale Power Generation and Transport Fuels Applications. Princeton University, Princeton, NJ.
Williams, R.H. 1994b. Photovoltaic Technologies. Princeton University, Princeton, NJ.
Wisniewski, J. and A.E. Lugo (Eds.). 1992. Natural Sinks of CO2 Kluwer Academic Publishers, Boston.
Data Errors, Corrections and Disclaimer
CIESIN follows procedures designed to ensure that data disseminated in
CIESIN's Host are of reasonable quality. If, despite these procedures,
users encounter apparent misstatements in CIESIN's Host, they should
contact CIESIN Customer Services at 517/797-2614 or via Internet e-mail
at CIESIN.Info@ciesin.org. CIESIN will notify the original data provider of the apparent errors or misstatements, and will attempt to correct any errors or misstatements. Neither CIESIN nor NASA verifies or guarantees the accuracy,
reliability, or completeness of the data provided.
Text Browser Utilities:
[CIESIN Home Page,
CIESIN Gateway,
Compass,
User Services,
Register,
FAQs,
Full-Text Search]